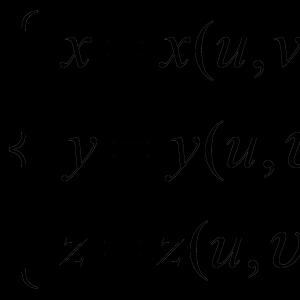Tangent plane and surface normal. Plane Tangent to Surface Normal and Tangent Planes to Surface

Download from Depositfiles

4. THEORY OF SURFACES.

4.1 EQUATIONS OF SURFACES.

A surface in 3D space can be defined:

1) implicitly: F ( x , y , z ) =0 (4.1)

2) explicitly: z = f ( x , y ) (4.2)

3) parametrically: (4.3)

or:
(4.3’)

where are scalar arguments
sometimes called curvilinear coordinates. For example, a sphere
it is convenient to set in spherical coordinates:
.

4.2 TANGENT PLANE AND NORMAL TO THE SURFACE.

If the line lies on the surface (4.1), then the coordinates of its points satisfy the surface equation:

Differentiating this identity, we get:

(4.4)

or
(4.4 ’ )

at every point on the curve on the surface. Thus, the gradient vector at non-singular points of the surface (at which the function (4.5) is differentiable and
) is perpendicular to the tangent vectors to any lines on the surface, i.e. can be used as a normal vector to formulate the equation of the tangent plane at point M 0 (x 0 , y 0 , z 0 ) surfaces

(4.6)

and as a direction vector in the normal equation:

(4.7)

In the case of an explicit (4.2) assignment of the surface, the equations of the tangent plane and the normal, respectively, take the form:

(4.8)

and
(4.9)

In the parametric representation of the surface (4.3), the vectors
lie in the tangent plane and the equation of the tangent plane can be written as:

(4.10)

and their vector product can be taken as the directing normal vector:

and the normal equation can be written as:

(4.11)

where
- parameter values corresponding to point M 0 .

In what follows, we confine ourselves to considering only those points of the surface where the vectors

are not equal to zero and are not parallel.

Example 4.1 Compose the equations of the tangent plane and the normal at the point M 0 (1,1,2) to the surface of the paraboloid of revolution
.

Solution: Since the paraboloid equation is given explicitly, according to (4.8) and (4.9) we need to find
at point M 0 :

, and at the point M 0
. Then the equation of the tangent plane at the point M 0 will take the form:

2(x -1)+2(y -1)-(z-2)=0 or 2 x +2 y -z - 2=0, and the normal equation
.

Example 4.2 Compose the equations of the tangent plane and the normal at an arbitrary point on the helicoid
, .

Solution. Here ,

Tangent plane equation:

Normal equations:

4.3 THE FIRST QUADRATIC FORM OF THE SURFACE.

If the surface is given by the equation

then the curve
on it can be given by the equation
(4.12)

Radius vector differential
along the curve corresponding to the displacement from the point M 0 to a nearby point M, is equal to

(4.13)

Because
is the differential of the curve arc corresponding to the same displacement), then

(4.14)

where .

The expression on the right side of (4.14) is called the first quadratic form of the surface and plays a huge role in the theory of surfaces.

Integrating differentialds ranging from t 0 (corresponds to point M 0 ) to t (corresponds to point M), we obtain the length of the corresponding segment of the curve

(4.15)

Knowing the first quadratic form of the surface, one can find not only the lengths, but also the angles between the curves.

If du , dv are the differentials of curvilinear coordinates corresponding to an infinitesimal displacement along one curve, and
— on the other hand, then, taking into account (4.13):

(4.16)

Using the formula

(4.17)

the first quadratic form makes it possible to calculate the area of a region
surfaces.

Example 4.3 On a helicoid, find the length of the helix
between two points.

Solution. Because on a helix
, then . Find at a point
the first quadratic form. Denoting andv = t , we obtain the equation of this helix in the form . Quadratic shape:

= - the first quadratic form.

Here . In formula (4.15) in this case
and arc length:

4.4 SECOND QUADRATIC FORM OF THE SURFACE.

Denote
- unit normal vector to the surface
:

(4.18) . (4.23)

A line on a surface is called a line of curvature if its direction at each point is the principal direction.

4.6 THE CONCEPT OF GEODETIC LINES ON THE SURFACE.

Definition 4.1 . A curve on a surface is called a geodesic if its principal normal is at every point where the curvature is nonzero, coincides with the normal to the surface.

Through each point of the surface in any direction passes, and only one geodesic. On a sphere, for example, great circles are geodesics.

A parametrization of a surface is called semi-geodesic if one family of coordinate lines consists of geodesics and the other is orthogonal to it. For example, on the sphere meridians (geodesics) and parallels.

A geodesic on a sufficiently small segment is the shortest among all curves close to it connecting the same points.

At some point and has continuous partial derivatives at it, at least one of which does not vanish, then in the vicinity of this point the surface given by equation (1) will be correct surface.

In addition to the above implicit way of setting surface can be defined clearly, if one of the variables, for example z, can be expressed in terms of the others:

Also exists parametric assignment method. In this case, the surface is determined by the system of equations:

The concept of a simple surface

More accurately, plain surface is the image of a homeomorphic mapping (that is, a one-to-one and mutually continuous mapping) of the interior of the unit square. This definition can be given an analytical expression.

Let a square be given on a plane with a rectangular coordinate system u and v , the coordinates of the interior points of which satisfy the inequalities 0< u < 1, 0 < v < 1. Гомеоморфный образ квадрата в пространстве с прямоугольной системой координат х, у, z задаётся при помощи формул х = x(u, v), у = y(u, v), z = z(u, v) (параметрическое задание поверхности). При этом от функций x(u, v), y(u, v) и z(u, v) требуется, чтобы они были непрерывными и чтобы для различных точек (u, v) и (u", v") были различными соответствующие точки (x, у, z) и (x", у", z").

An example simple surface is a hemisphere. The whole area is not plain surface. This necessitates a further generalization of the concept of a surface.

A subset of space in which each point has a neighborhood that is plain surface, is called correct surface .

Surface in differential geometry

Helicoid

catenoid

The metric does not uniquely determine the shape of the surface. For example, the metrics of a helicoid and a catenoid , parameterized in an appropriate way, coincide, that is, there is a correspondence between their regions that preserves all lengths (isometry). Properties that are preserved under isometric transformations are called internal geometry surfaces. The internal geometry does not depend on the position of the surface in space and does not change when it is bent without tension and compression (for example, when a cylinder is bent into a cone).

Metric coefficients determine not only the lengths of all curves, but in general the results of all measurements inside the surface (angles, areas, curvature, etc.). Therefore, everything that depends only on the metric refers to the internal geometry.

Normal and normal section

Normal vectors at surface points

One of the main characteristics of a surface is its normal- unit vector perpendicular to the tangent plane at a given point:

The sign of the normal depends on the choice of coordinates.

The section of a surface by a plane containing the normal (at a given point) forms a certain curve on the surface, which is called normal section surfaces. The main normal for a normal section coincides with the normal to the surface (up to a sign).

If the curve on the surface is not a normal section, then its principal normal forms an angle θ with the surface normal. Then the curvature k curve is related to curvature k n normal section (with the same tangent) Meunier's formula:

The coordinates of the normal vector for different ways of specifying the surface are given in the table:

	Normal coordinates at a surface point
implicit assignment
explicit assignment
parametric task

Curvature

For different directions at a given point on the surface, a different curvature of the normal section is obtained, which is called normal curvature; it is assigned a plus sign if the main normal of the curve goes in the same direction as the normal to the surface, or a minus sign if the directions of the normals are opposite.

Generally speaking, at every point on the surface there are two perpendicular directions e 1 and e 2 , in which the normal curvature takes the minimum and maximum values; these directions are called main. An exception is the case when the normal curvature is the same in all directions (for example, near a sphere or at the end of an ellipsoid of revolution), then all directions at a point are principal.

Surfaces with negative (left), zero (center), and positive (right) curvature.

Normal curvatures in principal directions are called principal curvatures; let's denote them by κ 1 and κ 2 . Size:

K= κ 1 κ 2

called Gaussian curvature, full curvature or simply curvature surfaces. There is also the term curvature scalar, which implies the result of convolution of the curvature tensor ; in this case, the curvature scalar is twice as large as the Gaussian curvature.

The Gaussian curvature can be calculated in terms of the metric, and therefore it is an object of the intrinsic geometry of surfaces (note that the principal curvatures do not belong to the intrinsic geometry). By the sign of curvature, you can classify the points of the surface (see figure). The curvature of the plane is zero. The curvature of a sphere of radius R is everywhere equal to . There is also a surface of constant negative curvature - a pseudosphere.

Geodesic lines, geodesic curvature

The curve on the surface is called geodetic line, or simply geodetic, if at all its points the main normal to the curve coincides with the normal to the surface. Example: on a plane, geodesics will be straight lines and line segments, on a sphere - great circles and their segments.

Equivalent definition: for a geodesic line, the projection of its main normal onto the contiguous plane is the zero vector. If the curve is not a geodesic, then the specified projection is nonzero; its length is called geodesic curvature k g curve on the surface. There is a relation:

where k is the curvature of this curve, k n- curvature of its normal section with the same tangent.

Geodesic lines refer to internal geometry. We list their main properties.

One and only one geodesic passes through a given point on the surface in a given direction.
On a sufficiently small area of the surface, two points can always be connected by a geodesic, and moreover, only one. Explanation: on a sphere, opposite poles are connected by an infinite number of meridians, and two close points can be connected not only by a segment of a large circle, but also by its addition to a full circle, so that uniqueness is observed only in a small one.
The geodesic is the shortest. More strictly: on a small piece of the surface, the shortest path between given points lies along the geodesic.

Square

Another important attribute of the surface is its square, which is calculated by the formula:

A surface is defined as a set of points whose coordinates satisfy a certain type of equation:

F (x , y , z) = 0 (1) (\displaystyle F(x,\,y,\,z)=0\qquad (1))

If the function F (x , y , z) (\displaystyle F(x,\,y,\,z)) is continuous at some point and has continuous partial derivatives at it, at least one of which does not vanish, then in the neighborhood of this point the surface given by equation (1) will be correct surface.

In addition to the above implicit way of setting, the surface can be defined clearly, if one of the variables, for example, z, can be expressed in terms of the others:

z = f (x , y) (1 ′) (\displaystyle z=f(x,y)\qquad (1"))

More strictly, plain surface is the image of a homeomorphic mapping (that is, a one-to-one and mutually continuous mapping) of the interior of the unit square. This definition can be given an analytical expression.

An example simple surface is a hemisphere. The whole area is not plain surface. This necessitates a further generalization of the concept of a surface.

A subset of space in which each point has a neighborhood that is plain surface, is called correct surface .

Surface in differential geometry

Helicoid

catenoid

Metric coefficients E , F , G (\displaystyle E,\ F,\ G) determine not only the lengths of all curves, but in general the results of all measurements inside the surface (angles, areas, curvature, etc.). Therefore, everything that depends only on the metric refers to the internal geometry.

Normal and normal section

Normal vectors at surface points

One of the main characteristics of a surface is its normal- unit vector perpendicular to the tangent plane at a given point:

m = [ r u ′ , r v ′ ] | [ r u ′ , r v ′ ] | (\displaystyle \mathbf (m) =(\frac ([\mathbf (r"_(u)) ,\mathbf (r"_(v)) ])(|[\mathbf (r"_(u)) ,\mathbf (r"_(v)) ]|))).

The sign of the normal depends on the choice of coordinates.

The section of the surface by a plane containing the normal of the surface at a given point forms a certain curve, which is called normal section surfaces. The main normal for a normal section coincides with the normal to the surface (up to a sign).

If the curve on the surface is not a normal section, then its principal normal forms an angle with the surface normal θ (\displaystyle \theta ). Then the curvature k (\displaystyle k) curve is related to curvature k n (\displaystyle k_(n)) normal section (with the same tangent) Meunier's formula:

k n = ± k cos θ (\displaystyle k_(n)=\pm k\,\cos \,\theta )

The coordinates of the normal vector for different ways of specifying the surface are given in the table:

	Normal coordinates at a surface point
implicit assignment	(∂ F ∂ x ; ∂ F ∂ y ; ∂ F ∂ z) (∂ F ∂ x) 2 + (∂ F ∂ y) 2 + (∂ F ∂ z) 2 (\displaystyle (\frac (\left(( \frac (\partial F)(\partial x));\,(\frac (\partial F)(\partial y));\,(\frac (\partial F)(\partial z))\right) )(\sqrt (\left((\frac (\partial F)(\partial x))\right)^(2)+\left((\frac (\partial F)(\partial y))\right) ^(2)+\left((\frac (\partial F)(\partial z))\right)^(2)))))
explicit assignment	(− ∂ f ∂ x ; − ∂ f ∂ y ; 1) (∂ f ∂ x) 2 + (∂ f ∂ y) 2 + 1 (\displaystyle (\frac (\left(-(\frac (\partial f )(\partial x));\,-(\frac (\partial f)(\partial y));\,1\right))(\sqrt (\left((\frac (\partial f)(\ partial x))\right)^(2)+\left((\frac (\partial f)(\partial y))\right)^(2)+1))))
parametric task	(D (y , z) D (u , v) ; D (z , x) D (u , v) ; D (x , y) D (u , v)) (D (y , z) D (u , v)) 2 + (D (z , x) D (u , v)) 2 + (D (x , y) D (u , v)) 2 (\displaystyle (\frac (\left((\frac (D(y,z))(D(u,v)));\,(\frac (D(z,x))(D(u,v)));\,(\frac (D(x ,y))(D(u,v)))\right))(\sqrt (\left((\frac (D(y,z))(D(u,v)))\right)^(2 )+\left((\frac (D(z,x))(D(u,v)))\right)^(2)+\left((\frac (D(x,y))(D( u,v)))\right)^(2)))))

Here D (y , z) D (u , v) = | y u ′ y v ′ z u ′ z v ′ | , D (z , x) D (u , v) = | z u ′ z v ′ x u ′ x v ′ | , D (x, y) D (u, v) = | x u ′ x v ′ y u ′ y v ′ | (\displaystyle (\frac (D(y,z))(D(u,v)))=(\begin(vmatrix)y"_(u)&y"_(v)\\z"_(u) &z"_(v)\end(vmatrix)),\quad (\frac (D(z,x))(D(u,v)))=(\begin(vmatrix)z"_(u)&z" _(v)\\x"_(u)&x"_(v)\end(vmatrix)),\quad (\frac (D(x,y))(D(u,v)))=(\ begin(vmatrix)x"_(u)&x"_(v)\\y"_(u)&y"_(v)\end(vmatrix))).

All derivatives are taken at the point (x 0 , y 0 , z 0) (\displaystyle (x_(0),y_(0),z_(0))).

Curvature

Generally speaking, at every point on the surface there are two perpendicular directions e 1 (\displaystyle e_(1)) and e 2 (\displaystyle e_(2)), in which the normal curvature takes the minimum and maximum values; these directions are called main. An exception is the case when the normal curvature is the same in all directions (for example, near a sphere or at the end of an ellipsoid of revolution), then all directions at a point are principal.

Surfaces with negative (left), zero (center), and positive (right) curvature.

Normal curvatures in principal directions are called principal curvatures; let's denote them κ 1 (\displaystyle \kappa _(1)) and κ 2 (\displaystyle \kappa _(2)). Size:

K = κ 1 κ 2 (\displaystyle K=\kappa _(1)\kappa _(2))

The graph of a function of 2 variables z = f(x,y) is a surface projected onto the XOY plane into the domain of the function D.
Consider the surface σ , given by the equation z = f(x,y) , where f(x,y) is a differentiable function, and let M 0 (x 0 ,y 0 ,z 0) be a fixed point on the surface σ , i.e. z0 = f(x0,y0). Appointment. The online calculator is designed to find tangent plane and surface normal equations. The decision is made in Word format. If you need to find the equation of the tangent to the curve (y = f(x)), then you need to use this service.

Function entry rules:

All variables are expressed in terms of x,y,z

Tangent plane to surface σ at her point M 0 is the plane in which the tangents to all curves drawn on the surface lie σ through a point M 0 .
The equation of the tangent plane to the surface given by the equation z = f(x,y) at the point M 0 (x 0 ,y 0 ,z 0) has the form:

z - z 0 \u003d f 'x (x 0, y 0) (x - x 0) + f ' y (x 0, y 0) (y - y 0)

The vector is called the surface normal vector σ at the point M 0 . The normal vector is perpendicular to the tangent plane.
Normal to the surface σ at the point M 0 is a straight line passing through this point and having the direction of the vector N.
The canonical equations of the normal to the surface given by the equation z = f(x,y) at the point M 0 (x 0 ,y 0 ,z 0), where z 0 = f(x 0 ,y 0), have the form:

Example #1. The surface is given by the equation x 3 +5y . Find the equation of the tangent plane to the surface at the point M 0 (0;1).
Solution. Let's write the tangent equations in general form: z - z 0 \u003d f "x (x 0, y 0, z 0) (x - x 0) + f" y (x 0, y 0, z 0) (y - y 0 )
By the condition of the problem x 0 = 0, y 0 = 1, then z 0 = 5
Find the partial derivatives of the function z = x^3+5*y:
f" x (x, y) = (x 3 +5 y)" x = 3 x 2
f" x (x, y) = (x 3 +5 y)" y = 5
At the point M 0 (0.1), the values of partial derivatives:
f"x(0;1) = 0
f" y (0; 1) = 5
Using the formula, we obtain the equation of the tangent plane to the surface at point M 0: z - 5 = 0(x - 0) + 5(y - 1) or -5 y + z = 0

Example #2. The surface is given implicitly y 2 -1/2*x 3 -8z. Find the equation of the tangent plane to the surface at the point M 0 (1;0;1).
Solution. We find partial derivatives of the function. Since the function is given in an implicit form, we are looking for derivatives by the formula:

For our function:

Then:

At the point M 0 (1,0,1) the values of partial derivatives:
f "x (1; 0; 1) \u003d -3 / 16
f"y(1;0;1) = 0
Using the formula, we obtain the equation of the tangent plane to the surface at point M 0: z - 1 \u003d -3 / 16 (x - 1) + 0 (y - 0) or 3 / 16 x + z- 19 / 16 \u003d 0

Example. Surface σ given by the equation z= y/x + xy – 5x 3 . Find the equation of the tangent plane and normal to the surface σ at the point M 0 (x 0 ,y 0 ,z 0) belonging to it if x 0 = –1, y 0 = 2.
Let's find the partial derivatives of the function z= f(x,y) = y/x + xy – 5x 3:
f x '( x,y) = (y/x + xy – 5x 3)' x \u003d - y / x 2 + y – 15x 2 ;
f y' ( x,y) = (y/x + xy – 5x 3)' y = 1/x + x.
Dot M 0 (x 0 ,y 0 ,z 0) belongs to the surface σ , so we can calculate z 0 , substituting the given x 0 = -1 and y 0 = 2 into the surface equation:

z= y/x + xy – 5x 3

z 0 = 2/(-1) + (–1) 2 – 5 (–1) 3 = 1.
At the point M 0 (–1, 2, 1) values of partial derivatives:
f x '( M 0) = –1/(-1) 2 + 2 – 15(–1) 2 = –15; fy'( M 0) = 1/(-1) – 1 = –2.
Using formula (5), we obtain the equation of the tangent plane to the surface σ at the point M 0:
z – 1= –15(x + 1) – 2(y – 2) z – 1= –15x – 15 – 2y + 4 15x + 2y + z + 10 = 0.
Using formula (6), we obtain the canonical equations of the normal to the surface σ at the point M 0:

.
Answers: tangent plane equation: 15 x + 2y + z+ 10 = 0; normal equations:

Example #1. Given a function z \u003d f (x, y) and two points A (x 0, y 0) and B (x 1, y 1). Required: 1) calculate the value z 1 of the function at point B; 2) calculate the approximate value z 1 of the function at point B based on the value z 0 of the function at point A, replacing the increment of the function during the transition from point A to point B with a differential; 3) compose the equation of the tangent plane to the surface z = f(x,y) at the point C(x 0 ,y 0 ,z 0).
Solution.
We write the tangent equations in general form:
z - z 0 \u003d f "x (x 0, y 0, z 0) (x - x 0) + f" y (x 0, y 0, z 0) (y - y 0)
According to the condition of the problem x 0 = 1, y 0 = 2, then z 0 = 25
Find the partial derivatives of the function z = f(x,y)x^2+3*x*y*+y^2:
f "x (x, y) \u003d (x 2 +3 x y + y 2)" x \u003d 2 x + 3 y 3
f "x (x, y) \u003d (x 2 +3 x y + y 2)" y \u003d 9 x y 2
At the point M 0 (1.2), the values of partial derivatives:
f" x (1; 2) = 26
f" y (1; 2) = 36
Using the formula, we obtain the equation of the tangent plane to the surface at the point M 0:
z - 25 = 26(x - 1) + 36(y - 2)
or
-26x-36y+z+73 = 0

Example #2. Write the equations of the tangent plane and the normal to the elliptical paraboloid z = 2x 2 + y 2 at the point (1;-1;3).

Definition. A point lying on a second-order surface given by the general equation (1) with respect to the ODSC is called non-singular if among the three numbers: there is at least one that is not equal to zero.

Thus, a point lying on a second-order surface is not singular if and only if it is its center, otherwise, when the surface is conical, and the point is the vertex of this surface.

Definition. A tangent to a second-order surface at a given non-singular point on it is a straight line passing through this point, intersecting the second-order surface at a double point, or being a rectilinear generatrix of the surface.

Theorem 3. The tangent lines to a second-order surface at a given non-singular point on it lie in the same plane, called the tangent plane to the surface at the point under consideration. The tangent plane equation has

Proof. Let , , be parametric equations of a straight line passing through a non-singular point of the second-order surface given by equation (1). Substituting into equation (1) , , instead of , , , we get:

Since the point lies on the surface (1), we also find from equation (3) (this value corresponds to the point ). In order for the point of intersection of the line with the surface (1) to be double, or for the line to lie entirely on the surface, it is necessary and sufficient that the equality be satisfied:

If at the same time:

Then the point of intersection of the straight line with the surface (1) is double. And if:

Then the line lies entirely on the surface (1).

From relations (4) and , , it follows that the coordinates , , of any point lying on any tangent to the surface (1) satisfy the equation:

Conversely, if the coordinates of some point other than satisfy this equation, then the coordinates , , of the vector satisfy relation (4), which means that the line is tangent to the surface under consideration.

Since the point is a non-singular point of the surface (1), then among the numbers , , there is at least one that is not equal to zero; so equation (5) is an equation of the first degree with respect to . This is the equation of the plane tangent to the surface (1) at a nonsingular point given on it.

Based on the canonical equations of second-order surfaces, it is easy to compose the equations of tangent planes to an ellipsoid, hyperboloid, etc. at a given point on them.

one). Tangent plane to ellipsoid:

2). Tangent plane to one and two-sheeted hyperboloids:

3). Tangent plane to elliptic and hyperbolic paraboloids:

§ 161. Intersection of a tangent plane with a surface of the second order.

We take a non-singular point of the surface of the second order as the origin of coordinates of the ODSC, the axis and place it in the plane tangent to the surface at the point . Then in the general equation of the surface (1) the free term is equal to zero: , and the equation of the plane touching the surface at the origin should look like: .

But the equation of the plane passing through the origin has the form: .

And, since this equation must be equivalent to the equation , then , , .

So, in the chosen coordinate system, the surface equation (1) should look like:

Conversely, if , then equation (6) is the equation of the surface passing through the origin of coordinates , and the plane is the tangent plane to this surface at the point . The equation of the line along which the tangent plane to the surface at a point intersects the surface (6) has the form:

If . This is an invariant in invariant theory for second-order lines. Equation (7)

This is the second line. By the form of this line, the invariant is , therefore:

For , here are two imaginary intersecting lines.

When - two real intersecting lines.

If , but at least one of the coefficients , , is not equal to zero, then the line of intersection (7) is two coinciding lines.

Finally, if , then the plane

is part of the given surface, and the surface itself breaks up, therefore, into a pair of planes

§ 162. Elliptic, hyperbolic or parabolic points of a surface of the second order.

1. Let the tangent plane to the surface of the second order at a point intersect it along two imaginary intersecting straight lines. In this case, the point is called an elliptical point of the surface.

2. Let the tangent plane to the surface of the second order at a point intersect it along two real lines intersecting at the point of contact. In this case, the point is called a hyperbolic point of the surface.

3. Let the tangent plane to the surface of the second order at a point intersect it along two coinciding straight lines. In this case, the point is called a parabolic point of the surface.

Theorem 4. Let the second-order surface with respect to the ODSC be given by equation (1) and this equation (1) be the equation of a real non-decaying surface of the second order. Then if ; then all points of the surface are elliptic.

Proof. Let us introduce a new coordinate system , choosing any non-singular point of the given surface as the origin of coordinates and placing the axes and in the plane tangent to the surface at the point . Equation (1) in the new coordinate system is transformed to the form:

Where . Let us calculate the invariant for this equation .

Since the sign does not change during the transition from one ODSC to another, the signs and are opposite, therefore, if , then ; and, as follows from the classification (see § 161), the tangent plane to the surface at a point intersects the surface along two imaginary intersecting lines, i.e. is an elliptical point.

2) A one-sheeted hyperboloid and a hyperbolic paraboloid consist of hyperbolic points.

3) The real cone of the second order (the vertex is excluded), elliptic (real), hyperbolic and parabolic cylinders consist of parabolic points.

parabolic cylinder.

To determine the location of a parabolic cylinder, it is enough to know:

1) a plane of symmetry parallel to the generators of the cylinder;

2) a tangent plane to the cylinder, perpendicular to this plane of symmetry;

3) a vector perpendicular to this tangent plane and directed towards the concavity of the cylinder.

If the general equation defines a parabolic cylinder, it can be rewritten as:

Let's pick m so that the plane

would be mutually perpendicular:

With this value m plane

will be a plane of symmetry parallel to the generators of the cylinder.

Plane

will be the tangent plane to the cylinder, perpendicular to the indicated plane of symmetry, and the vector

will be perpendicular to the found tangent plane and directed towards the concavity of the cylinder.